EMPTY RELATIONS

Concept and References

A manifestation of life via the always changing relations of elements that become building blocks of our reality, streams of information that define these relations. A situation that is felt subconsciously, a dynamic artwork without an artist. Always active, always morphing and constantly changing its reach.

Network illustrations are usually based on information. Widths, densities, geometric properties, these always communicate specific information. These images are perceived however as fairly abstract and random, in a way as something to do with the logic of glitches. This project is a manifestation of this misperception. It is random, it is abstract, it does not communicate information, it communicates the complexity of interdependence and interconnectivity. This new space should be treated as an inspiration for data art, where in this situation, data is not the point of focus, but the random and vague form generated itself.

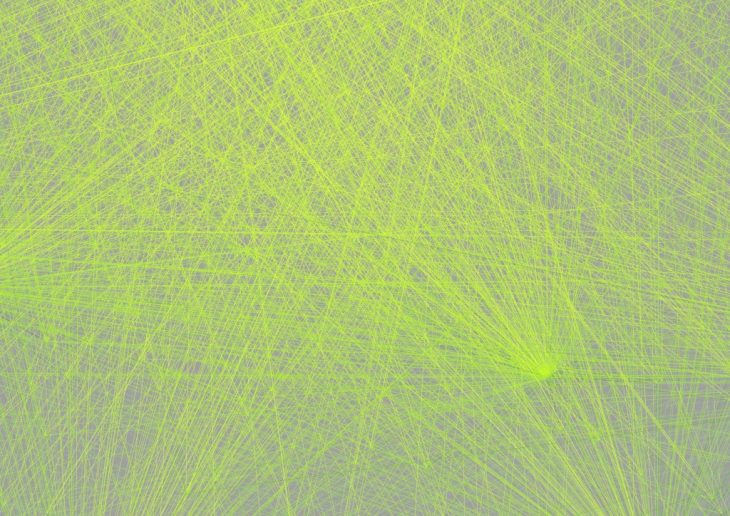
Evolution

There are two actions major groups of actions that generate this geometry; sphere character operations and interaction input.The first one deals with aspects of which the aim is to generate The sphere and its “roundness”. In this situation, the sphere is Made of points, do the higher density of points (population) the rounder it will become. The radius is also crucial, or rather its Relation with the amount of population points. Some other factors Of this group will be discussed later. The second group of input information is the relation with another Sphere. The second geometry dematerializes our primary One by subtracting its points population depending on where it is placed. These two processes seem distant and unrelated, however together, Give an interesting and rich experience of the geometry.

Grasshopper Operations

The grasshopper steps are quite simple and transparent, operations include various transformations, including list operations and Euclidean. The input modification area is visible on the left, and further processes are on the right.

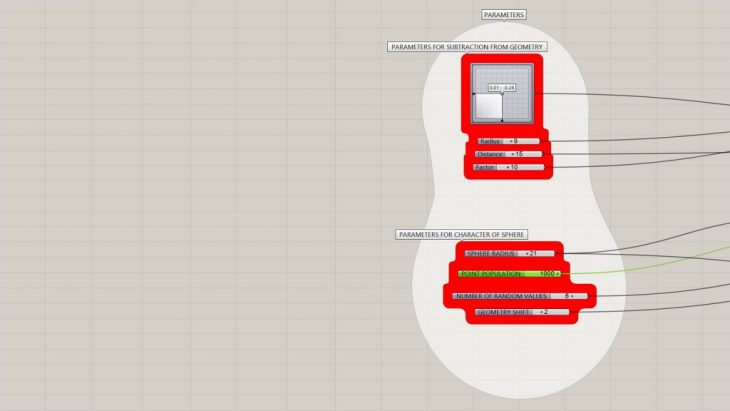
In the are of input at the top we have the infromation modification Of the second sphere used for subtraction of points from The prime sphere, basing on its location, as visible, these operations Include point location movement, rdius of sphere, distance Of affection, and a factor of force of this subtraction. In the lower area we have operations connected to the character Of the sphere; radius, point population (amount), random Major point picking value and a geometry shift.

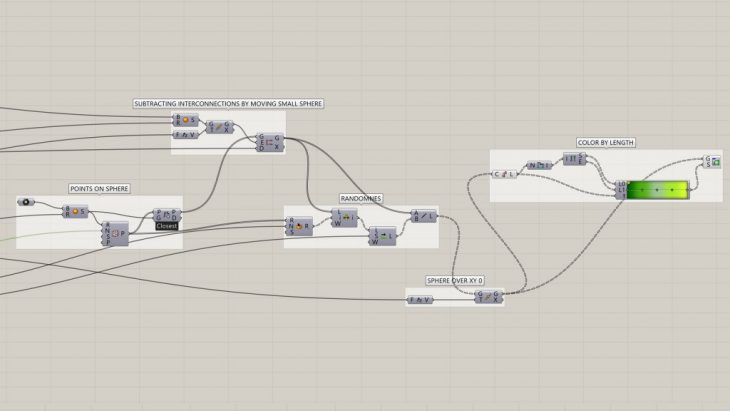
This side deals with executing functions and includes; -placing points on sphere, subtracting points from sphere by Movement of small second sphere, randomness factor, sphere Height(so it doesn’t cross xy plain), and gradient by length.

Conclusions and Future “debugging”

The result was very satisfactory however in the future I wish to modify the lines more efficiently and operate with their widths, making them dependent of length. Also a crucial problem had to do with baking this geometry. With every try the computer would freeze and in the only successful “bake” it released a series of lines, loosing their gradient. In the future i wish also to 3d print this geometry, as it is a good test for the limitations of a 3d printer.

Marcel Dawid